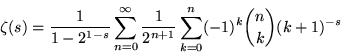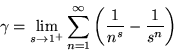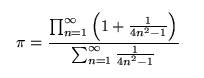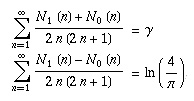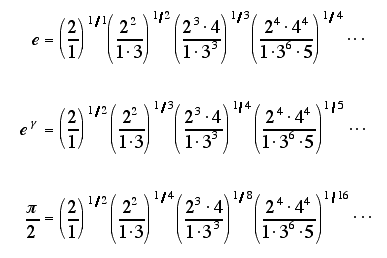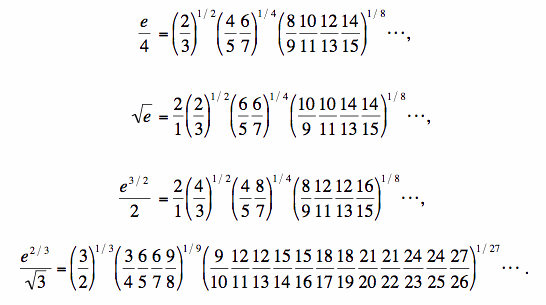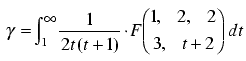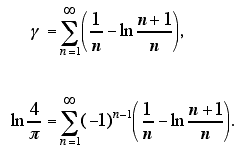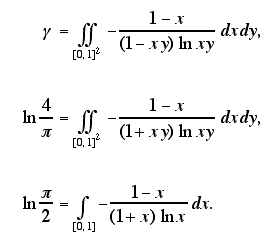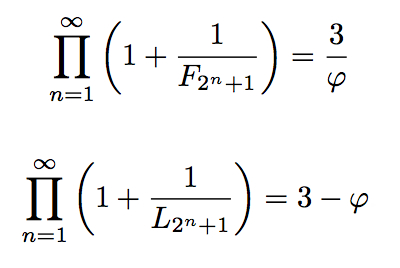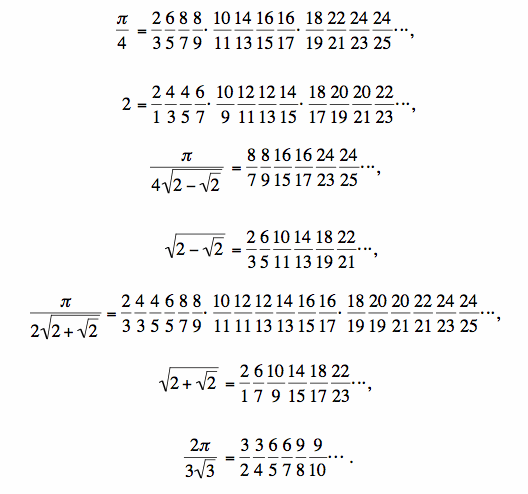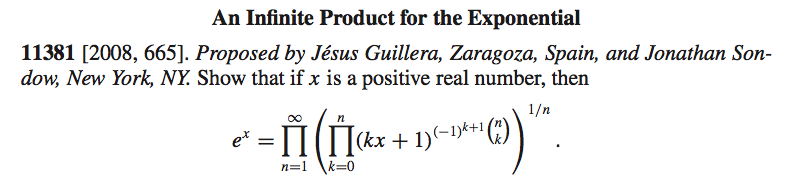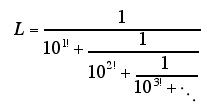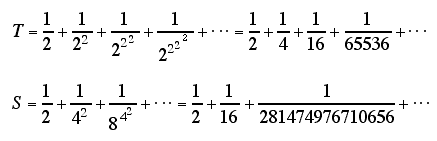|
|

|
| Before |
|

|
| Giving a talk at a meeting of the |
|

|
| After |
|
| MILNOR WINS THE 2004 STEELE PRIZE FOR EXPOSITION |

|
|
| FOOTNOTE TO MILNOR'S "DIFFERENTIAL TOPOLOGY 46..." |
|
|
My "nice" and "new" contributions to the Online Encyclopedia of Integer Sequences
NEW AND NOTEWORTHY 18 December 2018
* arXiv versions of [63, 74], Zbl reviews of [63, 70], online version of [71], new papers [38, 69, 72, 73, 74]
|
* Designation of [6] as the 6th most-cited Monthly paper on pi in [xlxvi]'s list of ``pi-star'' papers
|
* Co-author links in [53] to a tribute page for, and in [59] to a paper by, my friend and coauthor Sylvester
Reese, who passed away 21 May 2014
|
*
Link in [54] to Hajja's Zentralblatt review: "The paper under review is
a major contribution to the literature
on the arbelos. For the first
time, someone dares make drastic changes on the Archimedean
configuration..."
|
* Link in [xxxv] to Paul Loya's book "Amazing and Aesthetic Aspects of Analysis." My symmetric formula for pi
(see below) is a "beautiful expression" and "quite astonishing!"
|
*
Belated publication of my solution [21] to my Monthly Problem 11222: An
Infinite Product Based
on a Base; the editor comments
"The proposer's elegant solution covers all cases simultaneously and
efficiently."
|
* Link [xxvi] to Michel Waldschmidt's Bombay lectures on irrationality; he mentions my work on Euler's constant
[5] and gives my geometric proof that e is irrational and my irrationality measure for e [14]
|
* At long last, the MAA has put a citation of [3] in Dunham's book "Euler, the Master of Us All", and PUP has
cited [3] in Havil's book "Gamma: Exploring Euler's Constant" - see [I] and [III] below
|
* Link [xviii] to John Baez's web page This Week's Finds in Mathematical Physics. He displays the infinite products
for e, e^gamma,
and pi/2 shown below, calls them "eerily similar" and a "mystery thrown
down to us by the
math gods, like a bone from on
high", and cites my Monthly note "A faster product for pi ..." [12].
|
* Link [VII] to A. V. Zhukov's book El Omnipresente Número "Pi". My symmetric formula for pi (see below) is an
exhibit in his "museum of elegant mathematics."
|
|
FORMULAS AND PLOTS FROM MY WORK
(SCROLL DOWN FOR CITATIONS AND LINKS TO PAPERS)
| Conjectured by Knopp and first proved by Hasse |
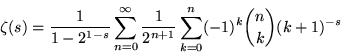
|
| A global series rediscovered in [1], [12]; cited in [iii], [viii], [III], [IV], [VIII], [IX] |
|
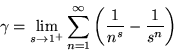
|
| Antisymmetric formula for Euler's constant [3], cited in [i], [iv], [I], [II], [III], [VI] |
|
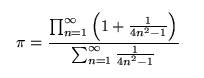
|
| Symmetric formula for pi [22], cited in [vi], [xxxv], [VII] |
|
|
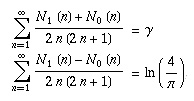
|
| Series with the number of 1s and 0s in the binary expansion of n, from [17], [18], cited in [xxxii] |
|
| |
The product below for e is due to
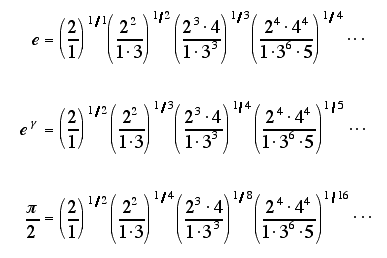
|
| Infinite products from [11], [12], cited in [iv], [xviii], [xxiii]; the 2nd found earlier by J. Ser |
|
| Partial products of Wallis's product (left) and my faster product for pi/2, from [12] |
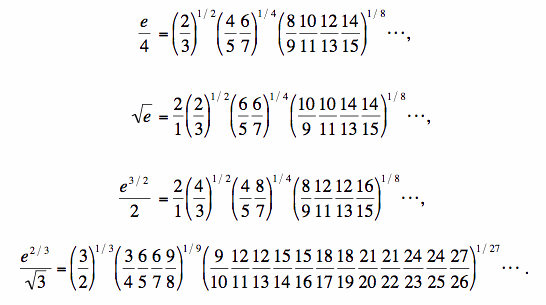
|
| Catalan-type infinite products, from [37] with Yi HUANG |
|
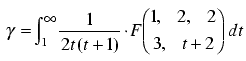
|
| Hypergeometric formula for Euler's constant [8], [11] |
|
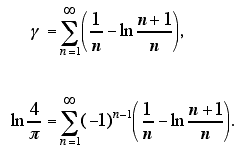
|
| Series showing ln(4/pi) is an "alternating Euler constant" [6], [17], [23], cited in [iv], [xxxii] |
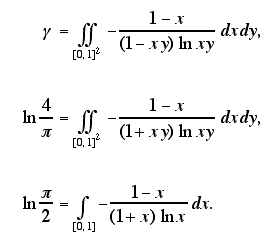
|
| Integrals from [6], [12], cited in [i], [iv], [XI]. The 3rd is a special case of Kummer's integral. |
|
| |
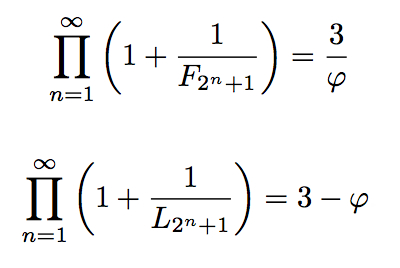
|
| Fibonacci- & Lucas-number products from [48], where phi is the golden ratio |
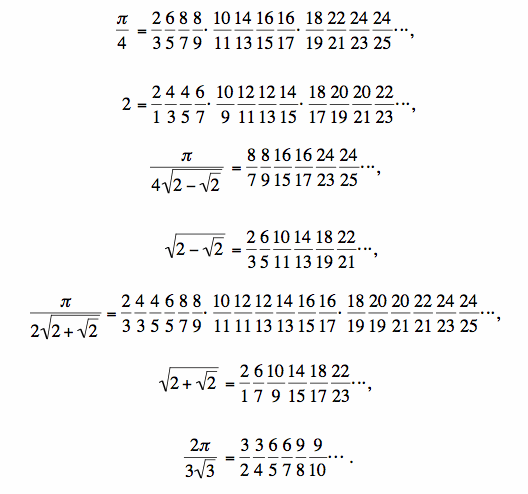
|
| Wallis-type infinite products, from [37] with Yi HUANG |
|
|
| Monthly Problem: a new binomial coefficient identity [19], [5] |
|
| Monthly Problem: inequalities for generalized binomial coefficients, from [9] and [20] |

|
| Monthly Problem: a new base-B generalization of the Woods-Robbins product (B=2), from [21] and [28] |
|
| Monthly Problem: Beukers-type double integral = Nesterenko-type series [5], [8], [10], [30], [VI] |
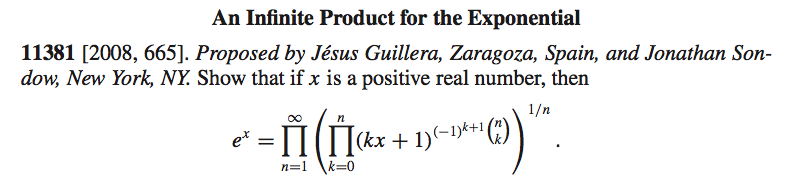
|
| Monthly Problem: An infinite product for e^x [31], from [16] |

|
| Monthly Problem: the highest power of 2 dividing a power sum [46], from [42], cited in [xxxiii] |
|
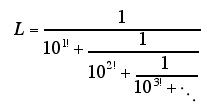
|
| The continued fraction L is a Liouville number with irrationality base one [10] |

|
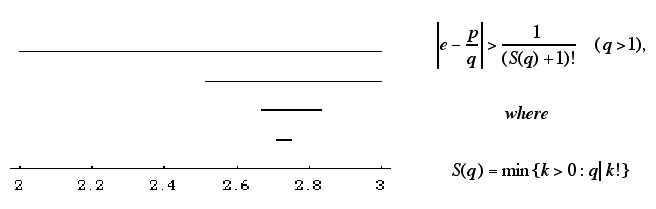
| A number with irrationality base k/h > 1, and an inequality for all integers p,q with q large [13] |
|
|
| A geometric proof that e is irrational, and a new measure of its irrationality [14] |
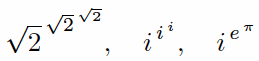
|
| Transcendental numbers if Schanuel's Conjecture holds, from [40] with Diego MARQUES, cited in [xlii] |
|
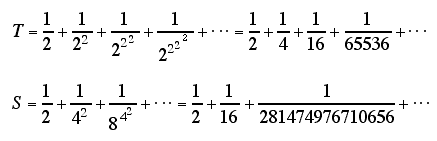
|
| T has irrationality base 2 and S has irrationality base infinity [10], [13] |
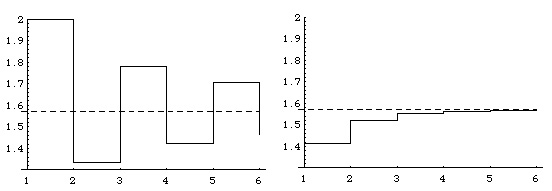
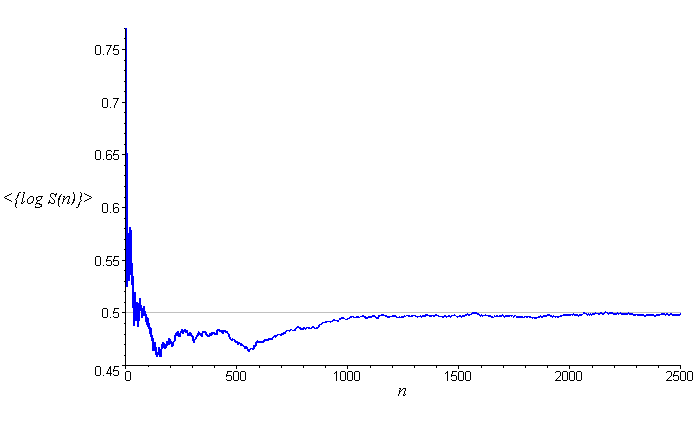
FOR THE THREE PLOTS BELOW I THANK
|
| If <{log S(n)}> does NOT tend to zero as n increases, then Euler's constant is irrational [5], [8] |
|
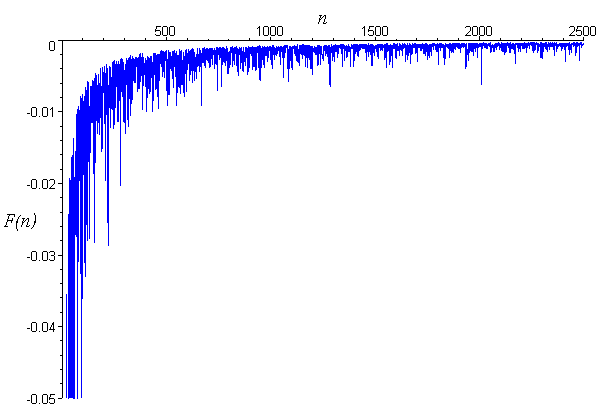
| If F(n) -> 0, then Euler's constant is irrational, but is not a super Liouville number [10] |
|
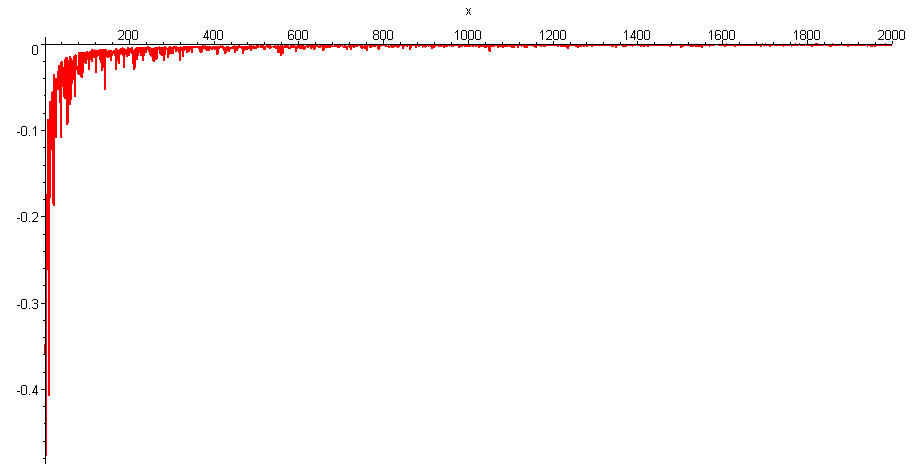
| If the graph -> 0 as n increases, then ln(pi) is irrational, but isn't a super Liouville number [13] |
SOME WEB PAGES AND ARTICLES CITING MY WORK IN NUMBER THEORY AND
GEOMETRY
|
i. X. Gourdon and P. Sebah's
|
Numbers, Constants and Computation/GammaFormulas(PDF)
|
|
ii. Matthew Watkins'
|
Inexplicable Secrets of Creation/RHreformulations
|
|
iii. Eric Weisstein's
|
|
|
iv. _____________
|
|
|
v. _____________
|
|
|
vi. _____________
|
|
|
vii. ____________
|
|
|
viii. Philippe Biane, Jim Pitman and Marc Yor's
|
|
|
ix. Wadim Zudilin's
|
Zeta Values on the Web
|
|
x. Michel Waldschmidt's
|
|
|
xi. American Scientist's
|
|
|
xii. Keith Matthews'
|
|
|
xiii. ____________
|
|
|
xiv. Neil J. Sloane's
|
|
|
xv. ____________
|
|
|
xvi. ___________
|
|
|
xvii. ___________
|
|
|
xviii. John Baez's
|
|
|
xix. Stefan Kraemer's
|
|
|
xx. Michel Waldschmidt's
|
|
|
xxi. _______________
|
|
|
xxii. _______________
|
|
|
xxiii. Jesus Guillera's
|
|
|
xxiv. Michel Waldschmidt's
|
|
|
xxv. _______________
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
xxxi. Thomas and Joseph Dence's
|
|
xxxii. Jean-Paul Allouche's
|
|
xxxiii. Doron Zeilberger's
|
|
|
|
|
|
|
|
|
|
|
xxxvii. Yuri Matiyasevich, Filip Saidak, and Peter Zvengrowski's
|
|
| |
|
xxxviii. Robert P. Schneider's
xxxix. Aliza Steurer and Thomas Hagedorn's
|
|
|
|
|
|
|
Errata and Addenda to Mathematical Constants, pp. 1, 4, 6, 53, 59, 64, 69, 71, 117, 125
|
xliii. Emmanuel Tsukerman's
|
|
xliv. B. Berndt, S. Kim, and A. Zaharescu's
|
|
|
|
|
|
|
|
xlvii. Antonio M. Oller-Marcén's
|
|
xlix. I. Ben-Ari, D. Hay, and A. Roitershtein's
xlx. Michel Waldschmidt's
xlxii. Yuri Matiyasevich's
xlxiii. B. Berndt, S. Kim, and A. Zaharescu's
xlxiv. B. Berndt, S. Kim, M. Phaovibul, and A. Zaharescu's
xlxvi. J. M. Borwein and S. T. Chapman's
xlxvii. Jacques Gelinas's
xlxviii. Jacques Gelinas's
|
|
SOME BOOKS CITING MY WORK IN NUMBER THEORY
|
I.
|
Euler, the Master of Us All
|
by William Dunham, MAA, 1999 (8th printing, 2010), p. 36 (my antisymmetric
formula for Euler's constant exhibits "a delightful symmetry")
|
|
|
|
Book of the Year 1967, p. 503
|
|
|
|
by Jonathan Borwein, Alf van der Poorten, Jeffrey Shallit, Wadim Zudilin, CUP, 2014, pp. 159, 205
|
|
| |
1. Analytic continuation of Riemann's zeta function and values at negative integers via Euler's transformation
of series, Proc. Amer. Math. Soc. 120 (1994) 421-424.
|
|
2. The Riemann Hypothesis, simple zeros, and the asymptotic convergence degree of improper Riemann sums, Proc.
Amer. Math. Soc. 126 (1998) 1311-1314.
|
|
3. An antisymmetric formula for Euler's constant, Math. Mag. 71 (1998) 219-220.
|
|
4. Zeros of the alternating zeta function on the line R(s)=1, Amer. Math. Monthly 110 (2003) 435-437. Chinese
translation published in: Mathematical Advance in Translation, Chinese Academy of Sciences, 33 #2 (2014) 188-189.
|
|
5. Criteria for irrationality of Euler's constant, Proc. Amer. Math. Soc. 131 (2003) 3335-3344.
|
|
6. Double integrals for Euler's constant and ln 4/pi and an analog of Hadjicostas's formula, Amer.
Math. Monthly 112 (2005) 61-65.
|
|
7. Formulas for pi(x) and the nth prime, with
. . .
|
|
|
|
|
8. A hypergeometric approach, via linear forms involving logarithms, to criteria for irrationality of Euler's
constant, Math. Slovaca 59 (2009) 1-8, with an Appendix by . . .
|
|
Sergey ZLOBIN
|
|
9. Euler's constant, q-logarithms, and formulas of Ramanujan and Gosper, Ramanujan
J. 12 (2006) 225-244, with . . .
|
Wadim ZUDILIN
|
|
10. An irrationality measure for Liouville numbers and conditional measures for Euler's constant (2003,
e-print).
|
|
11. An infinite product for e^gamma via hypergeometric formulas for Euler's constant, gamma (2003, e-print).
|
|
12. A faster product for pi and a new integral for ln pi/2, Amer. Math. Monthly
112 (2005) 729-734 (article), 113 (2006) 670 (addendum).
|
|
13. Irrationality measures, irrationality bases, and a theorem of Jarnik (2004, e-print).
|
Abstract, PDF
|
14. A geometric proof that e is irrational and a new measure of its irrationality, Amer.
Math. Monthly 113 (2006) 637-641 (article), 114 (2007) 659 (addendum).
|
|
15. Corrigendum: On the irrationality of some alternating series, Studia
Univ. Babes-Bolyai Math. 49 no. 1 (2004) 105-106, with . . .
|
Jozsef SANDOR
|
|
16. Double integrals and infinite products for some classical constants via analytic continuations of Lerch's
transcendent, Ramanujan J. 16 (2008) 247-270, with . . .
|
|
|
17. New Vacca-type rational series for Euler's constant and its "alternating" analog ln 4/pi,
|
|
|
| |
|
| |
18. Summation of series defined by counting blocks of digits, J. Number Theory
123 (2007) 133-143, with . . .
|
|
Jeffrey SHALLIT
|
|
19. Problem 11026 (see image above): An identity involving harmonic numbers, Amer.
Math. Monthly 110 (2003) 636 (proposal), 112 (2005) 367-369 (solution).
|
|
My solution
|
|
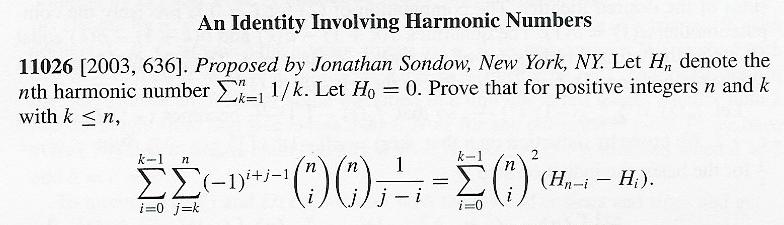
20. Problem 11132 (see image above): Choice bounds, Amer. Math. Monthly 112 (2005) 180 (proposal), 114
(2007) 359-360 (solution).
|
|
|
| |
|
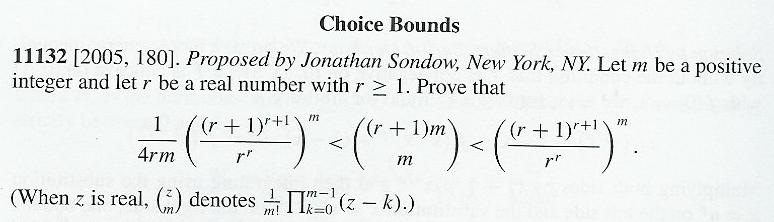
21. Problem 11222 (see image above): An infinite product based on a base, Amer.
Math. Monthly 113 (2006) 459 (proposal), 115 (2008) 954-955 (solution).
|
|
My solution
|
|
| |
22. Problem 88 (see image above): A symmetric formula for pi, Math Horizons 5 (Sept., 1997) 32, 34.
|
23. The generalized-Euler-constant function gamma(z) and a generalization of Somos's quadratic recurrence
constant, J. Math. Anal. Appl. 332 (2007) 292-314, with . . .
|
|
Petros HADJICOSTAS
|
|
24. The Taylor series for e and the primes 2, 5, 13, 37, 463: a surprising connection (2006, e-print).
|
PDF
|
25. Integrals over polytopes, multiple zeta values and polylogarithms, and Euler's constant, Math.
Notes (in English) 84 (2008) pp. 568-583, Erratum p. 887; Mat. Zametki (in Russian) 84:4 (2008)
pp. 609-626; with . . .
|
Sergey ZLOBIN
|
|
26. A simple counterexample to Havil's "reformulation" of the Riemann Hypothesis, Elemente
der Mathematik 67 (2012) 61-67.
|
|
27. Which partial sums of the Taylor series for e are convergents to e? (and a link to the primes 2, 5,
13, 37, 463). Part II (includes corrected version of Part I), with . . .
|
Kyle SCHALM
|
|
28. Infinite products with strongly B-multiplicative exponents, Annales
Univ.
Sci. Budapest, Sect.
Comp. 28 (2008) 35-53 (article), 32 (2010) 253 (errata), with . . .
|
|
|
29. Primes, pi, and irrationality measure (2007, e-print).
|
Abstract, PDF
|
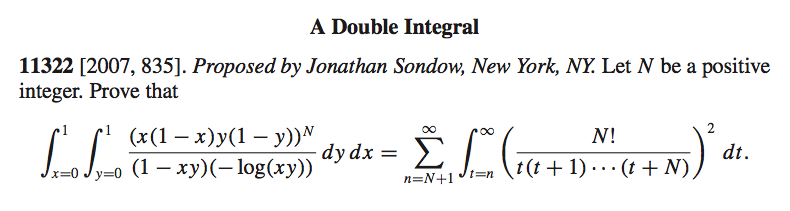
30. Problem 11322 (see image above): A double integral, Amer. Math. Monthly 114 (2007) 835 (proposal),
116 (2009) 650 (solution).
|
|
31. Problem 11381 (see image above): An infinite product for the exponential, Amer.
Math. Monthly, 115
(2008) 665 (proposal), 117 (2010) 283-284 (solution), with . . .
|
|
|
32. Ramanujan primes and Bertrand's postulate, Amer. Math. Monthly 116
(2009) 630-635.
|
|
33. Reducing the Erdos-Moser equation 1^n + 2^n + … + k^n = (k+1)^n modulo k and k^2, Integers 11 (2011) article A34, with . . .
|
|
|
34. A monotonicity property of Riemann's xi function and a reformulation of the Riemann Hypothesis, Periodica
Mathematica Hungarica 60 (2010) 37-40, with . . .
|
|
|
35. Algebraic and transcendental solutions of some exponential equations, Annales
Mathematicae et Informaticae 37 (2010) 151-164, with . . .
|
|
|
|
36. How not to prove/disprove the Riemann Hypothesis (in preparation), with . . .
|
|
|
37. New Wallis- and Catalan-type infinite products for pi, e, and sqrt(2+sqrt(2)), Amer.
Math. Monthly 117 (2010) 912-917, with . . .
|
|
|
38. Initial sums of the Legendre symbol: Is min + max ≥ 0 ?, with . . .
|
|
|
39. Lerch quotients, Lerch primes, Fermat-Wilson quotients, and the Wieferich-non-Wilson primes 2, 3,
14771,
|
|
|
40.
Schanuel's conjecture and algebraic powers z^w and w^z with z and w transcendental, East-West Journal
of Mathematics 12, no. 1 (2010) 75-84, with . . .
|
|
|
41. Proofs
of power sum and
binomial coefficient congruences via Pascal's identity, Amer. Math. Monthly
118 (2011) 549-551, with . . .
|
|
|
42.
Divisibility of power sums and the generalized Erdos-Moser equation, Elemente der Mathematik 67 (2012) 182–186,
with . . .
|
|
|
43.
Ramanujan primes: bounds, runs, twins, and gaps, J. Integer Seq. 14 (2011) article 11.6.2, with . . .
|
|
|
|
44. Robin's theorem, primes, and a new elementary reformulation of the Riemann Hypothesis, Integers
11 (2011) article A33, with . . .
|
|
|
Jean-Louis NICOLAS
|
|
45.
The Schanuel subset conjecture implies Gelfond's power tower conjecture (2012, e-print), with . . .
|
|
|
46. Problem 11546 (see image above): 2-adic Valuation of Bernoulli-style Sums, Amer. Math. Monthly 118 (2011) 84 (proposal), 119 (2012) 886-887 (solution), with . . .
|
|
47. From the Monthly over 100 years ago … Halsted on Gauss, Amer. Math. Monthly
121 (2014) p. 963.
|
|
48. Evaluation of Tachiya's algebraic infinite products involving Fibonacci and Lucas numbers,
|
|
|
49. Generalized Ramanujan primes, with Olivia BECKWITH, Ryan RONAN, and . . .
|
|
|
|
|
|
50. On SA, CA, and GA numbers, Ramanujan J. 29 (2012) 359-384, with . . .
|
|
|
Jean-Louis NICOLAS
|
|
|
51. Translation of D. Zimin’s Dynasty Foundation and Pierre Deligne Contests for Young Mathematicians, Russian
Math. Surveys 62:1 (2007) 213–216.
|
|
52. Rebuttal of Kowalenko's paper as concerns the irrationality of Euler's
constant, Acta Applicandae Mathematicae 121 (2012) 1-3, with . . .
|
|
Online Preview
|
|
53. Universal parabolic constant, MathWorld
and Online Encyclopedia of Integer Sequences, 2005, with . . .
|
|
|
54. The parbelos, a parabolic analog of the arbelos, Amer. Math. Monthly 120 (2013) 929-935.
|
|
55. New approximations to Euler's constant (in preparation), with . . .
|
|
56. On variations of the arbelos (in preparation), with . . .
|
|
57. Ramanujan, Robin, highly composite numbers, and the Riemann Hypothesis, with
. . .
|
Jean-Louis NICOLAS
|
Ramanujan 125 (Gainesville, FL, 2012), 145-156, Contemp. Math., 627, Amer. Math. Soc., Providence, RI, 2014.
|
|
58. The p-adic order of power sums, the Erdos-Moser equation, and Bernoulli numbers (2014,
e-print), with
|
|
Abstract, PDF
|
59. Universal equilateral hyperbolic constant, Online Encyclopedia of Integer Sequences, 2013, with . . .
|
|
|
60. More circles in the generalized arbelos (in preparation), with . . .
|
|
61. Primitive antiharmonic numbers, Online Encyclopedia of Integer Sequences, 2013, with . . .
|
|
|
62. On the congruence 1^m + 2^m + … + m^m == n (mod m) with n | m, Monatsh.
Math. 177 (2015) 421-436, with . . .
|
|
|
|
|
63. Primary pseudoperfect numbers, arithmetic progressions, and the Erdos-Moser equation, Amer.
Math. Monthly 124 (2017) 232-240, with . . .
|
|
|
64. On Pascal's triangle (in preparation), with . . .
|
|
|
|
|
65. Ideal multigrades, symmetric and non-symmetric: the Prouhet-Tarry-Escott problem, Online Encyclopedia of Integer Sequences, 2014.
|
|
66. Summation of rational series twisted by strongly B-multiplicative coefficients, with
. . .
|
|
|
|
67. A parameterized series representation of Apery's constant zeta(3), with . . .
|
|
|
|
68. Irrationality and transcendence of alternating series via continued fractions (in
preparation)
|
|
|
69. Newton quadrilaterals, the associated cubic equations, and their rational solutions, Amer. Math.
Monthly, forthcoming, with . . .
|
|
70. Power-sum denominators, Amer. Math. Monthly 124 (2017) 695-709, with . . .
|
|
|
71. The denominators of power sums of arithmetic progressions, Integers 18 (2018) article A95, with .
. .
|
|
|
72. Hypergeometry of the parbelos, Amer. Math. Monthly, forthcoming, with . . .
|
|
John CAMPBELL
|
73. On the interplay between hypergeometric functions, complete elliptic integrals, and Fourier-Legendre expansions,
submitted, with . . .
|
|
John CAMPBELL
|
Abstract, PDF
|
74. Extending Babbage’s (non-)primality tests
|
|
|
|
|
MY PAPERS IN TOPOLOGY, NUMBER THEORY & GEOMETRY:
|